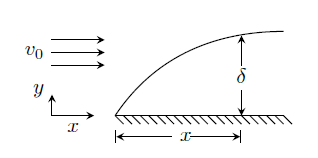
GATE-CH-2013-11-fm-1mark

The mass balance for a fluid with density (\(\rho \)) and velocity vector (\(\vec {V}\)) is

• \(\displaystyle \frac {\partial \rho }{\partial t} + \nabla \cdot \left (\rho \vec {V} \right ) = 0 \)

• \(\displaystyle \frac {\partial \rho }{\partial t} + \vec {V}\cdot \left (\nabla \rho \right ) = 0 \)

• \(\displaystyle \frac {\partial \rho }{\partial t} + \rho \left (\nabla \cdot \vec {V} \right ) = 0 \)

• \(\displaystyle \frac {\partial \rho }{\partial t} - \vec {V}\cdot \left (\nabla \rho \right ) = 0 \)

GATE-ME-2015-S2-58-fm-2mark

Match the following pairs:

Equation	Physical Interpretation
P. \(\nabla \times \vec{V}=0\)	I. Incompressible continuity equation
Q. \(\nabla \cdot \vec{V}=0\)	II. Steady flow
R. \(\dfrac{D\vec{V}}{Dt}=0\)	III. Irrotational flow
S. \( \dfrac{\partial\vec{V}}{\partial t} = 0 \)	IV. Zero acceleration of fluid particle

      

• P-IV, Q-I, R-II, S-III

• P-IV, Q-III, R-I, S-II

• P-III, Q-I, R-IV, S-II

• P-III, Q-I, R-II, S-IV

GATE-CH-2003-34-fm-2mark

A fluid element has a velocity \(\vec {V} = -y^2x\vec {i} + 2yx^2\vec {j}\). The motion at \((x,y) = (1/\sqrt {2}, 1)\) is ____________

• rotational and incompressible

• rotational and compressible

• irrotational and compressible

• irrotational and incompressible

GATE-CH-2008-40-fm-2mark

A steady flow field of an incompressible fluid is given by \(\vec {V} = (Ax+By)\hat {i} - Ay\hat {j}\), where \(A=1\) s\(^{-1}\), \(B=1\) s\(^{-1}\), and \(x, y\) are in meters. The magnitude of the acceleration (in m/s²) of a fluid particle at \((1,2)\) is

• 1

• \(\sqrt {2}\)

• \(\sqrt {5}\)

• \(\sqrt {10}\)

GATE-CH-2009-31-fm-2mark

For an incompressible flow, the \(x\)- and \(y\)- components of the velocity vector are \[ v_x = 2(x+y); \quad v_y = 3(y+z) \] where \(x,y,z\) are in meters and velocities are in m/s. Then the \(z\)-component of the velocity vector \((v_z)\) of the flow for the boundary condition \(v_z=0\) at \(z=0\) is

• \(5z\)

• \(-5z\)

• \(2x+3z\)

• \(-2x-3z\)

GATE-CH-2010-19-fm-1mark

The stream function in a \(xy\)-plane is given below: \[ \psi = \frac {1}{2}x^2y^3 \] The velocity vector for this stream function is

• \(\displaystyle xy^3\vec {\imath} - \frac {3}{2}x^2y^2\vec {\jmath}\)

• \(\displaystyle \frac {3}{2}x^2y^2\vec {\imath} - xy^3\vec {\jmath}\)

• \(\displaystyle \frac {3}{2}x^2y^2\vec {\imath} + xy^3\vec {\jmath}\)

• \(\displaystyle xy^3\vec {\imath} + \frac {3}{2}x^2y^2\vec {\jmath}\)

GATE-ME-2009-31-fm-2mark

You are asked to evaluate assorted fluid flows for their suitability in a given laboratory application. The following three flow choices, expressed in terms of the two-dimensional velocity fields in the \(xy\)-plane, are made available. 

\[\begin {align*} \text {P.} \qquad & u =2y, \quad & v&=-3x \\ \text {Q.} \qquad & u=3xy,\quad & v&=0 \\ \text {R.} \qquad & u=-2x,\quad & v&=2y \end {align*} \]

Which flow(s) should be recommended when the application requires the flow to be incompressible and irrotational?

• P and R

• Q

• Q and R

• R

GATE-XE-2011-B-19-fm-2mark

A flow has a velocity field given by \[ \vec {v} = 2x\hat {\imath } - 2y\hat {\jmath } \] The velocity potential \(\phi (x,y)\) for the flow is

• \(2x-2y+\text { const.}\)

• \(2xy+\text { const.}\)

• \(x^2+y^2+\text { const.}\)

• \(x^2-y^2+\text { const.}\)

GATE-CH-2014-39-fm-2mark

An incompressible fluid is flowing through a contraction section of length \(L\) and has a 1-D (\(x\)-direction) steady state velocity distribution, \(\displaystyle u=u_0\left (1+\frac {2x}{L} \right )\). If \(u_0 = 2\) m/s and \(L = 3\) m, the convective acceleration (in m/s²) of the fluid at \(L\) is ____________

• Answer

GATE-CH-2014-48-fm-2mark

In a steady incompressible flow, the velocity distribution is given by \(\vec {v}=3x\hat {\imath }-Py\hat {\jmath }+5z\hat {k}\), where, \(v\) is in m/s and \(x, y\), and \(z\) are in m. In order to satisfy the mass conservation, the value of the constant \(P\) (in s\(^{-1}\)) is ____________

• Answer

GATE-CH-1992-13-b-fm-6mark

For flow over a flat plate where in a laminar boundary layer is present for the case of a zero pressure gradient, the parabolic velocity profile for velocity \(u\) is given by \[ \begin {align*} u &= a_1y + a_2y^2 \text { for } y \le \delta \\
u &= v_o \text { for } y \ge \delta \end {align*} \] Find \(a_1\) and \(a_2\).

• Answer

GATE-CH-2002-6-fm-5mark

Consider the flow in a liquid film of constant thickness (\(\delta\)) along a vertical wall as shown in figure below.

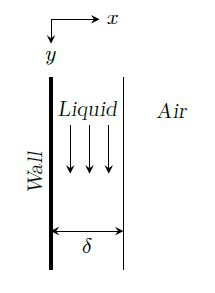
Assuming laminar, one-dimensional, fully developed flow, the \(y\)-direction Navier Stokes equation reduces to \[\mu \frac{d^2v_y}{dx^2} + \rho g = 0\] where \(v_y\) is the velocity in \(y\) direction, \(\mu\) is the viscosity and \(\rho\) is the density of the liquid.

1. State the boundary conditions to be used for the solution of velocity profile.

2. Solve for the velocity profile.

3. If \(Q\) is the volumetric flow rate per unit width of the wall, how is it related to the film thickness, \(\delta\).

• Answer